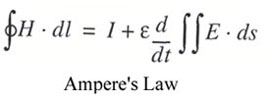Displacement Current
Displacement current is the complicated-looking last term in Ampère’s Law, the first of Maxwell’s Equation. It is this term that Maxwell added to Maxwell’s Equations and is the term that makes all the magic happen.
In Ampère’s Law the left hand side of the equation (that is, left of the equal sign) tells us to add up all the magnetic field, H, which is parallel to a closed curve--any curve--which we choose. The first term of the right hand side is the total electric current flowing through that same curve.
The last term (everything right of the plus sign) is just like the last term of Faraday’s Law, only it has to do with electric field, instead of magnetic field. The two integral signs (the S-like symbols) tell us to sum up all the electric field, E, flowing through a closed curve, the exact same curve we used for the left hand side of the equation.
Once more, as with the similar term in Faraday’s Law, we are not interested in the actual value of the total electric field that “jumps through the loop.” Rather, the d/dt tells us that we must calculate how fast the total electric field is changing. It could be a very large electric field, but if it stays the same all the time, it does not matter one bit. For this term, we are only interested in electric fields that change and the faster they change, the more important they are.
Notice that a changing electric field affects this equation in exactly the same way the current, I, does. Current needs a conductor, like copper, to flow. In contrast, a changing electric field has no problem “flowing” through perfect insulators. This is why it is called displacement current. If you know about the electronic component called capacitors, displacement current is what “flows” through the dielectric between the two plates.
Maxwell noticed that Faraday’s Law and Ampère’s Law are strikingly similar except that the original Ampère’s Law did not have this last term. So Maxwell made an educated guess and added this term to Ampère’s Law. Now the two laws would be almost exact mirror images of each other. While this was emotionally satisfying, did it really mean anything? Maxwell played with the equations to see what would happen. And what happened was magic.
The displacement current term says that a changing electric field (right hand side of Ampère’s Law, above) creates a magnetic field (left hand side of Ampère’s Law). Faraday’s Law says a changing magnetic field creates an electric field (note the symmetry?). So Maxwell wondered why couldn’t electric fields and magnetic fields, once they are set up correctly, just keep working back and forth? Each one changes and creates the other in turn, going back and forth like this, all by themselves with absolutely nothing else to help them along.
The answer, of course, is that is exactly what they do. The result is electromagnetic radiation. Various kinds of electromagnetic radiation include visible light (which you are using to read this), radio waves (used by your cell phone), microwaves (used in radar), x-rays (used in medicine), and so on.
Maxwell mathematically predicted that light was really electromagnetic radiation. However, fate decreed that it would not be until 1887, eight years after Maxwell’s death, that Heinrich Hertz would actually show experimentally that a new kind of wave, radio waves, were also electromagnetic. Maxwell’s prediction and Hertz’s subsequent experimental validation were one of the most stunning achievements of 19th century physics.